What is the Prisoners' Dilemma in Game Theory?
Origins of the Prisoners’ Dilemma
The Game Theory example that would eventually become known as the Prisoners’ Dilemma was first formulated by Melvin Dresher and Merrill Flood at the RAND Corporation in 1950. At a Stanford University seminar, Dr. Albert W. Tucker gave a more formal description of the “game” that included a story and actual payoffs. (Straffin, 1993)
Although the scenario for the Prisoners’ Dilemma has been presented in many ways, all basically describe the same situation. The following description of this significant example of a non-zero-sum game is one that is commonly used.
The Prisoners’ Dilemma Scenario
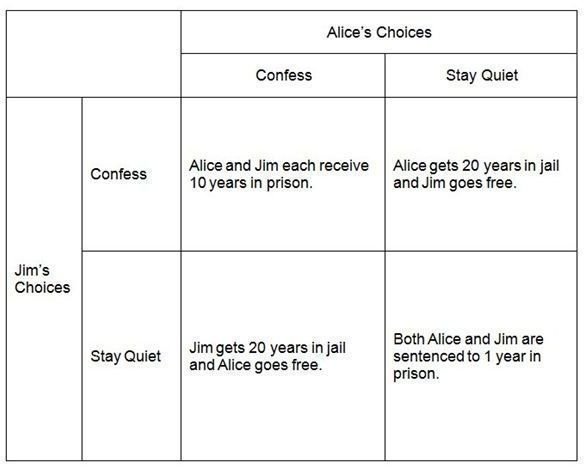
Suppose that Alice and Jim are both arrested and accused of stealing goods from a warehouse. The police separate Alice and Jim to question them and offer them each the same deal. However, Alice and Jim are not given any information on how the other responds to the police’s questions or offer.
What is the offer given to both Alice and Jim separately? Each one has the choice of keeping completely quiet about the crime or confessing on behalf of both parties. If both confess and implicate the partner, each one will receive a sentence of 10 years in prison. However, if both keep quiet, each one will only have to serve 1 year in prison. On the other hand, if one confesses and the other stays quiet, the one who confesses will go completely free while the other suspect who stays quiet will receive a 20-year prison sentence.
The possible outcomes are summarized in the following table. (Click the table for a larger view.)
Choosing the Best Course of Action
Why is the example of the Prisoners’ Dilemma so important? Well, if we assume that both Alice and Jim are only acting in their own self interests and that each one wants to spend the least amount of time in prison as possible, the best strategy for each individual is to confess. Why is this the case? It may help to think about the scenario in the following manner.
- If Jim confesses, Alice will receive 10 years in prison if she confesses and 20 years in prison if she stays quiet. So Alice’s best strategy for this situation is to confess.
- If Jim stays quiet, Alice will go free if she confesses. Alternatively, she would receive one year in prison if she stays quiet. So again, Alice’s best strategy is to confess.
- Since Jim only has two possible options and Alice’s best strategy for each of those options is to confess, she should confess regardless of what Jim chooses.
- The same logic holds true for Jim if he is evaluating his strategy by considering Alice’s possible options. Therefore, each prisoner, if acting individually, should confess.
But, wait a second! If both prisoners confess, then each one is going to have to spend the next 10 years in prison. If we look at the game as a whole, we see that this outcome is clearly dominated by the option in which each prisoner stays quiet – in this latter option, each one would only receive a one year sentence.
The Prisoners’ Dilemma is an example of a very important concept. If each player is acting individually, trying to make the best decision for himself or herself based on all known information, that decision doesn’t necessarily have to be the best decision for the players as a group (despite the fact that the decision is strategically sound). In other words, even though both players are rationally choosing the option that best serves their own self interests, the result is an outcome that is worse for both of them compared to other possible outcomes.
Applications in the Real World
There are actually many applications of the Prisoners’ Dilemma in the real world. One of the most commonly discussed involves resource conservation issues. That is, if all individuals are only looking out for themselves, the “best” solution (at least in the short term), is often to disregard conservation measures. However, if everyone does this, the result is generally detrimental to all parties concerned.
Another example lies in the realm of political science with the proliferation of nuclear weapons. The leaders of Country A may conclude that it is in the country’s best interest to continue to stockpile nuclear weapons based on the following arguments:
- If Country B is building more weapons, then Country A needs to make sure that it keeps up so that it will have bargaining power and won’t become a target for Country B’s weapons.
- If Country B isn’t accumulating more weapons, then Country A has the chance to “get ahead” of Country B, giving itself an advantage.
In actuality, if both countries would agree to produce fewer nuclear weapons, it’s possible that a greater advantage could be had by each one, both economically and socially.
References and Related Resources:
1. Straffin, Philip D. Game Theory and Strategy. Washington, DC: Mathematical Association of America, 1993.
2. Prisoner’s Dilemma, Stanford Encyclopedia of Philosophy.